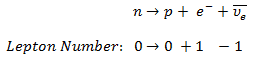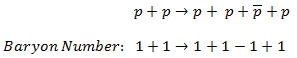What is Antimatter
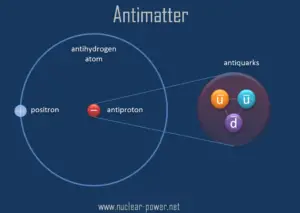 Antimatter is a term referring to material that would be made up of “antiatoms” in which antiprotons and antineutrons would form the nucleus around which positrons (antielectrons) would move. The term is also used for antiparticles in general.
Antimatter is a term referring to material that would be made up of “antiatoms” in which antiprotons and antineutrons would form the nucleus around which positrons (antielectrons) would move. The term is also used for antiparticles in general.
Antimatter particles bind with one another to form antimatter, just as ordinary particles bind to form normal matter. For example, a positron and an antiproton can form an antihydrogen atom. Physical principles indicate that complex antimatter atomic nuclei are possible, as well as anti-atoms corresponding to the known chemical elements.
Our visible Universe is almost entirely composed of matter, and very little antimatter has existed since the Big Bang. This problem is known as the baryon asymmetry. This asymmetry of matter and antimatter in the visible universe is one of the great unsolved problems in physics.
Particles and Antiparticles
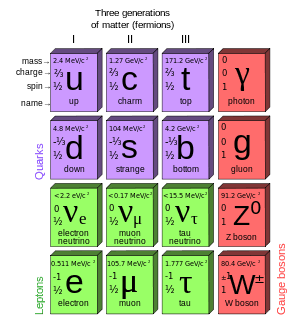
Quarks and electrons are some of the elementary particles. A number of fundamental particles have been discovered in various experiments. So many, that researchers had to organize them, just like Mendeleev did with his periodic table. This is summarized in a theoretical model (concerning the electromagnetic, weak, and strong nuclear interactions) called the Standard Model. In particle physics, an elementary particle or fundamental particle is a particle whose substructure is unknown, thus it is unknown whether it is composed of other particles.
In particle physics, corresponding to most kinds of particles there is an associated antiparticle. An antiparticle has the same mass and opposite charge (including electric charge).
For example, for every quark there is a corresponding type of antiparticle. The antiquarks have the same mass, mean lifetime, and spin as their respective quarks, but the electric charge and other charges have the opposite sign.
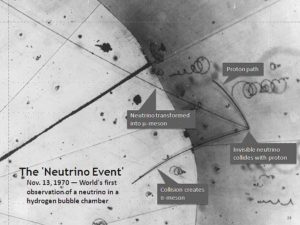
The original idea for antiparticles came from a relativistic wave equation developed in 1928 by the English scientist P. A. M. Dirac (1902-1984). He realised that his relativistic version of the Schrödinger wave equation for electrons predicted the possibility of antielectrons. These were discovered by Paul Dirac and Carl D. Anderson in 1932 and named positrons. They studied cosmic-ray collisions via a cloud chamber – a particle detector in which moving electrons (or positrons) leave behind trails as they move through the gas. Positron paths in a cloud chamber trace the same helical path as an electron but rotate in the opposite direction with respect to the magnetic field direction due to their having the same magnitude of charge-to-mass ratio but with opposite charge and, therefore, opposite signed charge-to-mass ratios. Although Dirac did not himself use the term antimatter, its use follows on naturally enough from antielectrons, antiprotons, etc.
It was predicted that other particles also would have antiparticles. In 1955 the antiparticle to the proton, the antiproton, which carries a negative charge, was discovered at the University of California, Berkeley. Since then, the antiparticles of many other subatomic particles have been created in particle accelerator experiments. On the other hand, a few, like the photon and π0, do not have distinct antiparticles. These particles are their own antiparticles.
Matter – Antimatter Creation and Annihilation
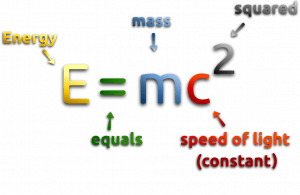 At the beginning of the 20th century, the notion of mass underwent a radical revision. Mass lost its absoluteness. One of the striking results of Einstein’s theory of relativity is that mass and energy are equivalent and convertible one into the other. Equivalence of the mass and energy is described by Einstein’s famous formula E = mc2. In words, energy equals mass multiplied by the speed of light squared.
At the beginning of the 20th century, the notion of mass underwent a radical revision. Mass lost its absoluteness. One of the striking results of Einstein’s theory of relativity is that mass and energy are equivalent and convertible one into the other. Equivalence of the mass and energy is described by Einstein’s famous formula E = mc2. In words, energy equals mass multiplied by the speed of light squared.
In special theory of relativity certain types of matter may be created or destroyed, but in all of these processes, the mass and energy associated with such matter remains unchanged in quantity. As a result of the conservation of lepton and baryon numbers, antimatter (antiparticles) can be created out of energy, but only if a particle counterpart for every antiparticle is created as well. It will be demonstrated in the following sections.
Matter – Antimatter Creation
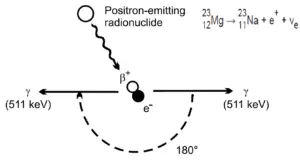
 Matter – Antimatter creation occurs naturally in high-energy processes involving cosmic rays, and also in high-energy experiments in accelerators in Earth. High-energy cosmic rays impacting Earth’s atmosphere (or any other matter in the Solar System) produce minute quantities of antiparticles in the resulting particle jets, which are immediately annihilated by contact with nearby matter. The presence of the resulting antimatter is detectable by the two gamma rays (with 511 keV) produced every time positrons annihilate with nearby matter.
Matter – Antimatter creation occurs naturally in high-energy processes involving cosmic rays, and also in high-energy experiments in accelerators in Earth. High-energy cosmic rays impacting Earth’s atmosphere (or any other matter in the Solar System) produce minute quantities of antiparticles in the resulting particle jets, which are immediately annihilated by contact with nearby matter. The presence of the resulting antimatter is detectable by the two gamma rays (with 511 keV) produced every time positrons annihilate with nearby matter.
Antimatter creation is also very common in nuclear decay of many isotopes. Let assume a decay of potassium-40. Naturally occurring potassium is composed of three isotopes, of which 40K is radioactive. Traces of 40K are found in all potassium, and it is the most common radioisotope in the human body. 40K is a radioactive isotope of potassium which has a very long half-life of 1.251×109 years and undergoes both types of beta decay.
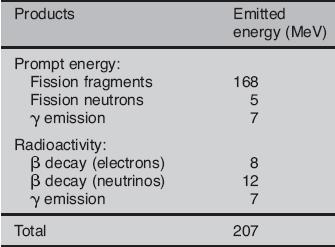
- About 89.28% of the time (10.72% is by electron capture), it decays to calcium-40 (40Ca) with emission of a beta particle (β−, an electron) with a maximum energy of 1.33 MeV and an antineutrino, which is an antiparticle to the neutrino.
- Very rarely (0.001% of the time) it will decay to 40Ar by emitting a positron (β+) and a neutrino.
Another very interesting source of antimatter is, in fact, a nuclear reactor. Nuclear reactors are the major source of human-generated antineutrinos. This is due to the fact that antineutrinos are produced in a negative beta decay. In a nuclear reactor occurs especially the β−decay, because the common feature of the fission fragments is an excess of neutrons. Please note that billions of solar neutrinos per second pass (mostly without any interaction) through every square centimeter (~6×1010) on the Earth’s surface and antineutrino radiation is by no means dangerous.
Finally, the fact is that antimatter is much more common, than it may seem.In January 2011, research by the American Astronomical Society discovered antimatter (positrons) originating above thunderstorm clouds. It is suggested that these positrons are formed in terrestrial gamma-ray flashes (TGF). These positrons are produced in gamma-ray flashes created by electrons accelerated by strong electric fields in the clouds. TGFs are brief bursts occurring inside thunderstorms and associated with lightning. The streams of positrons and electrons collide higher in the atmosphere to generate more gamma rays. About 500 TGFs may occur every day worldwide, but mostly go undetected.
Matter – Antimatter Annihilation
As was written, a particle and its antiparticle have the same mass as one another, but opposite electric charge, and other differences in quantum numbers. That means a proton has positive charge while an antiproton has negative charge and therefore they attract each other. A collision between any particle and its antiparticle partner is known to lead to their mutual annihilation. Since matter and antimatter carry an immense amount of energy (due to E = mc2), their mutual annihilation is associated with production of intense photons (gamma rays), neutrinos, and sometimes less-massive particle–antiparticle pairs.
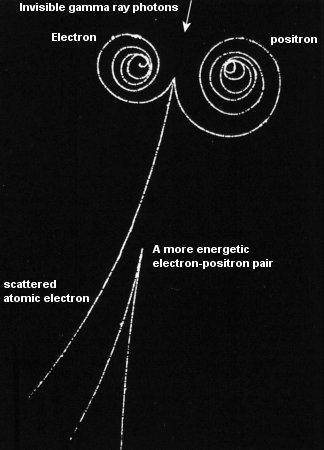
One of best known processes is electron-positron annihilation. Electron–positron annihilation occurs when a negatively charged electron and a positively charged positron collide.When a low-energy electron annihilates a low-energy positron (antiparticle of electron), they can only produce two or more photons (gamma rays). The production of only one photon is forbidden because of conservation of linear momentum and total energy. The production of another particle is also forbidden because of both particles (electron-positron) together do not carry enough mass-energy to produce heavier particles. When an electron and a positron collide, they annihilate resulting in the complete conversion of their rest mass to pure energy (according to the E=mc2 formula) in the form of two oppositely directed 0.511 MeV gamma rays (photons).
e− + e+ → γ + γ (2x 0.511 MeV)
This process must satisfy a number of conservation laws, including:
- Conservation of electric charge. The net charge before and after is zero.
- Conservation of linear momentum and total energy. T
- Conservation of angular momentum.
Matter and Antimatter – Laws of Conservation in Nuclear Reactions
In analyzing nuclear reactions, we apply the many conservation laws. Nuclear reactions are subject to classical conservation laws for charge, momentum, angular momentum, and energy (including rest energies). Additional conservation laws, not anticipated by classical physics, are are electric charge, lepton number and baryon number. Certain of these laws are obeyed under all circumstances, others are not.
At this point, we describe especially the relationship between antimatter and the following laws of conservation:
Law of Conservation of Lepton Number
 In particle physics, the lepton number is used to denote which particles are leptons and which particles are not. Each lepton has a lepton number of 1 and each antilepton has a lepton number of -1. Other non-leptonic particles have a lepton number of 0. The lepton number is a conserved quantum number in all particle reactions. A slight asymmetry in the laws of physics allowed leptons to be created in the Big Bang.
In particle physics, the lepton number is used to denote which particles are leptons and which particles are not. Each lepton has a lepton number of 1 and each antilepton has a lepton number of -1. Other non-leptonic particles have a lepton number of 0. The lepton number is a conserved quantum number in all particle reactions. A slight asymmetry in the laws of physics allowed leptons to be created in the Big Bang.
The conservation of lepton number means that whenever a lepton of a certain generation is created or destroyed in a reaction, a corresponding antilepton from the same generation must be created or destroyed. It must be added, there is a separate requirement for each of the three generations of leptons, the electron, muon and tau and their associated neutrinos.
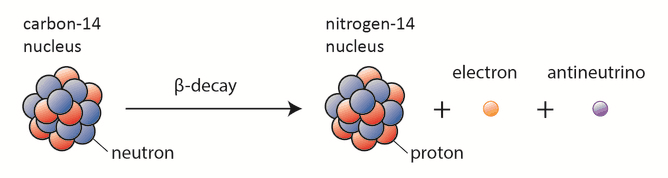
Consider the decay of the neutron. The reaction involves only first generation leptons: electrons and neutrinos:
Since the lepton number must be equal to zero on both sides and it was found that the reaction is a three-particle decay (the electrons emitted in beta decay have a continuous rather than a discrete spectrum), the third particle must be an electron antineutrino.
Law of Conservation of Baryon Number
In particle physics, the baryon number is used to denote which particles are baryons and which particles are not. Each baryon has a baryon number of 1 and each antibaryon has a baryon number of -1. Other non-baryonic particles have a baryon number of 0. Since there are exotic hadrons like pentaquarks and tetraquarks, there is a general definition of baryon number as:
where nq is the number of quarks, and nq is the number of antiquarks.
The baryon number is a conserved quantum number in all particle reactions.
The law of conservation of baryon number states that:
The sum of the baryon number of all incoming particles is the same as the sum of the baryon numbers of all particles resulting from the reaction.
For example, the following reaction has never been observed:
even if the incoming proton has sufficient energy and charge, energy, and so on, are conserved. This reaction does not conserve baryon number since the left side has B =+2, and the right has B =+1.
On the other hand, the following reaction (proton-antiproton pair production) does conserve B and does occur if the incoming proton has sufficient energy (the threshold energy = 5.6 GeV):
As indicated, B = +2 on both sides of this equation.
From these and other reactions, the conservation of baryon number has been established as a basic principle of physics.
This principle provides basis for the stability of the proton. Since the proton is the lightest particle among all baryons, the hypothetical products of its decay would have to be non-baryons. Thus, the decay would violate the conservation of baryon number. It must be added some theories have suggested that protons are in fact unstable with very long half-life (~1030 years) and that they decay into leptons. There is currently no experimental evidence that proton decay occurs.
Law of Conservation of Electric Charge
The law of conservation of electric charge can be demonstrated also on positron-electron pair production. Since a gamma ray is electrically neutral and sum of the electric charges of electron and positron is also zero, the electric charge in this reaction is also conserved.
Ɣ → e– + e+
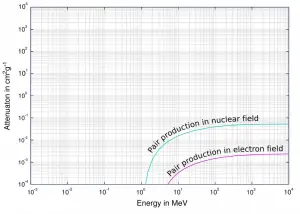
It must be added, in order for electron-positron pair production to occur, the electromagnetic energy of the photon must be above a threshold energy, which is equivalent to the rest mass of two electrons. The threshold energy (the total rest mass of produced particles) for electron-positron pair production is equal to 1.02MeV (2 x 0.511MeV) because the rest mass of a single electron is equivalent to 0.511MeV of energy. If the original photon’s energy is greater than 1.02MeV, any energy above 1.02MeV is according to the conservation law split between the kinetic energy of motion of the two particles. The presence of an electric field of a heavy atom such as lead or uranium is essential in order to satisfy conservation of momentum and energy. In order to satisfy both conservation of momentum and energy, the atomic nucleus must receive some momentum. Therefore a photon pair production in free space cannot occur.
We hope, this article, Antimatter, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about radiation and dosimeters.