What is Neutron Capture – Radiative Capture – Definition

Neutron Capture – Radiative Capture

The neutron capture is one of the possible absorption reactions that may occur. In fact, for non-fissionable nuclei it is the only possible absorption reaction. Capture reactions result in the loss of a neutron coupled with the production of one or more gamma rays. This capture reaction is also referred to as a radiative capture or (n, γ) reaction, and its cross-section is denoted by σ_γ.

The radiative capture is a reaction, in which the incident neutron is completely absorbed and compound nucleus is formed. The compound nucleus then decays to its ground state by gamma emission. This process can occur at all incident neutron energies, but the probability of the interaction strongly depends on the incident neutron energy and also on the target energy (temperature). In fact the energy in the center-of-mass system determines this probability.

Neutron Capture at Small Neutron Flux

It must be noted we have to distinguish between radiative captures at small neutron flux and at high neutron flux. At small neutron flux, as in a nuclear reactor, the compound nucleus has time to decay between two neutron captures. The most usual capture reactions that occur inside a power reactor are below:

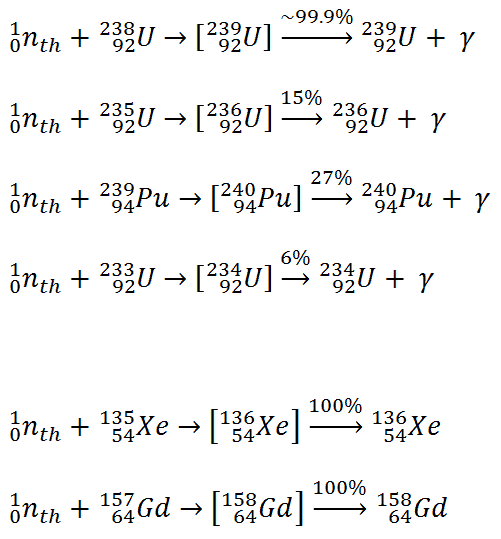
Neutron Capture at High Neutron Flux

At very high flux the atomic nuclei do not necessarily have enough time to decay via beta particle emission between neutron captures. This happens inside stars, where a really tremendous flux may be reached. As a result of many capture reactions without beta decay the mass number rises by a large amount, while the atomic number stays the same. Only afterwards, the highly unstable nuclei decay via many β− decays to stabilize itself.

Since the process entails a succession of many rapid neutron captures, it is called the r-process. The r-process is a nucleosynthesis process that occurs in core-collapse supernovae and is responsible for the creation of approximately half of the neutron-rich atomic nuclei heavier than iron.

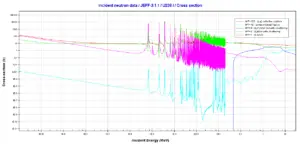Uranium 238. Comparison of cross-sections.Source: JANIS (Java-based Nuclear Data Information Software); The JEFF-3.1.1 Nuclear Data Library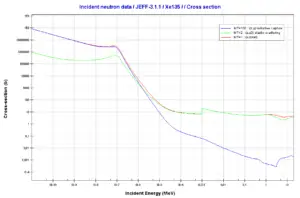Xenon – 135. Neutron absorption and scattering. Comparison of cross-sections.Source: JANIS (Java-based Nuclear Data Information Software); The JEFF-3.1.1 Nuclear Data LibraryHydrogen. Neutron absorption and scattering. Comparison of cross-sections.Source: JANIS (Java-based Nuclear Data Information Software); The JEFF-3.1.1 Nuclear Data Library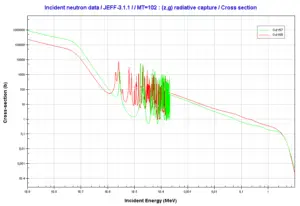Gadolinium 155 and 157. Comparison of radiative capture cross-sections.
Source: JANIS (Java-based Nuclear Data Information Software); The JEFF-3.1.1 Nuclear Data Library

Neutron Capture and Fuel Breeding

In reactor calculations, the neutron capture reaction is as important as the fission reaction. Its impact on the neutron balance is evident. But this reaction is of importance also from another point of view. Fissionable nuclei or even fissile nuclei may capture a neutron, this capture leads to formation of unstable nuclei with higher neutron number. Such unstable nuclei undergo a nuclear decay, which may lead to formation of another fissile nuclei. This process is also referred to as the nuclear transmutation and is responsible for new fuel breeding in nuclear reactors.

 

Radiative Capture Cross-section

The likelihood of a neutron radiative capture is represented by the radiative capture cross section as σ_γ. As usual, the cross-section can be divided into three regions according to the incident neutron energy.

• 1/v Region
• Resonance Region
• Fast Neutrons Region

1/v Region

In the common case, the cross section is usually much larger at low energies than at high energies. For thermal neutrons (in 1/v region), also radiative capture cross-sections increase as the velocity (kinetic energy) of the neutron decreases. Therefore the 1/v Law can be used to determine shift in capture cross-section, if the neutron is in equilibrium with a surrounding medium. This phenomenon is due to the fact the nuclear force between the target nucleus and the neutron has a longer time to interact.

Resonance Region

The largest cross-sections are usually at neutron energies, that lead to long-lived states of the compound nucleus. The compound nuclei of these certain energies are referred to as nuclear resonances and its formation is typical in the resonance region. The widths of the resonances increase in general with increasing energies. At higher energies the widths may reach the order of the distances between resonances and then no resonances can be observed. The narrowest resonances are usually compound states of heavy nuclei (such as fissionable nuclei).

Since the mode of decay of the compound nucleus does not depend on the way the compound nucleus was formed, the nucleus sometimes emits a gamma ray (radiative capture) or sometimes emits a neutron (scattering). In order to understand the way, how a nucleus will stabilize itself, we have to understand the behaviour of compound nucleus.

The compound nucleus emits a neutron only after one neutron obtains an energy in collision with other nucleon greater than its binding energy in the nucleus. It have some delay, because the excitation energy of the compound nucleus is divided among several nucleons. It is obvious the average time that elapses before a neutron can be emitted is much longer for nuclei with large number of nucleons than when only a few nucleons are involved. It is a consequence of sharing the excitation energy among a large number of nucleons.

This is the reason the radiative capture is comparatively unimportant in light nuclei but becomes increasingly important in the heavier nuclei.

The lifetime of a compound nucleus is inversely proportional to its total width. Narrow resonances therefore correspond to capture while the wider resonances are due to scattering.

Table of cross-sections.

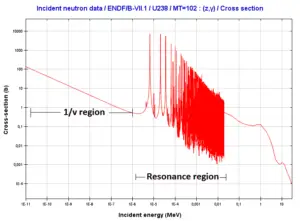Radiative Capture Cross-section – region of resonances of 238U nuclei.
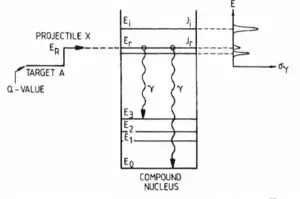
Source: JANIS (Java-based Nuclear Data Information Software); The JEFF-3.1.1 Nuclear Data Library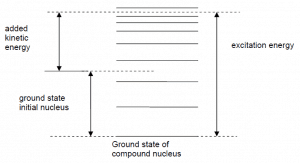Energy levels of compound state. For neutron absorption reaction on ²³⁸U the first resonance E1 corresponds to the excitation energy of 6.67eV. E0 is a base state of ²³⁹U.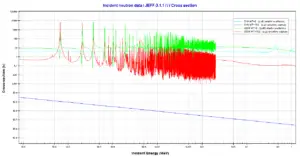Radiative capture and elastic scattering cross-sections in ¹⁶O and ²³⁸U.

Example:

Source: R. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983).

The first resonance in ²³⁸U at 6.67 eV, which corresponds to the first virtual level in ²³⁹U, has a total width of only 0.027 eV, and the mean life of this state is 2.4×10^-14s. By contrast, the resonance observed at 443 keV in ¹⁶O, which corresponds to the first virtual state in ¹⁷O, has a total width of 41 keV, giving a mean lifetime of 1.5×10^-21s. Thus it is highly likely that the compound state in ²³⁹U decays at least to some extent by gamma ray emission, while compound state in ¹⁷O must decay primarily by nucleon emission. The 443-keV resonance in ¹⁶O is clearly a scattering resonance, whereas the 6.67-eV resonance in ²³⁸U is in part a capture resonance.

Doppler Broadening

In general, Doppler broadening is the broadening of spectral lines due to the Doppler effect caused by a distribution of kinetic energies of molecules or atoms. In reactor physics a particular case of this phenomenon is the thermal Doppler broadening of resonances caused by the thermal motion of the target particle in the nuclear fuel.

The Doppler broadening of resonances is very important phanomenon, which improves reactor stability. The prompt temperature coefficient of most thermal reactors is negative, owing to an nuclear Doppler effect. Although the absorbtion cross-section depends significantly on incident neutron energy, the shape of the cross-section curve depends also on target temperature.

Nuclei are located in atoms which are themselves in continual motion owing to their thermal energy. As a result of these thermal motions neutrons impinging on a target appears to the nuclei in the target to have a continuous spread in energy. This, in turn, has an effect on the observed shape of resonance. The resonance becomes shorter and wider than when the nuclei are at rest.

Although the shape of a resonance changes with temperature, the total area under the resonance remains essentially constant. But this does not imply constant neutron absorbtion. Despite the constant area under resonance, a resonance integral, which determines the absorbtion, increases with increasing target temperature. This, of course, decreases coefficient k (negative reactivity is inserted).

Fast Neutrons Region

The radiative capture cross-section at energies above the resonance region drops rapidly to very small values. This rapid drop is caused by the compound nucleus, which is formed in more highly-excited states. In these highly-excited states it is more likely that one neutron obtains an energy in collision with other nucleon greater than its binding energy in the nucleus. The neutron emission becomes dominant and gamma decay becomes less important. Moreover, at high energies, the inelastic scattering and (n,2n) reaction are highly probable at the expense of both elastic scattering and radiative capture.

 

See also:

See also:

Neutron Reactions

See also: