Critical Energy – Threshold Energy for Fission
In principle, any nucleus, if brought into sufficiently high excited state, can be splitted. For fission to occur, the excitation energy must be above a particular value for certain nuclide. The minimum excitation energy required for fission to occur is known as the critical energy (Ecrit) or threshold energy.The critical energy depends on the nuclear structure and is quite large for light nuclei with Z < 90. For heavier nuclei with Z > 90, the critical energy is about 4 to 6 MeV for A-even nuclei, and generally is much lower for A-odd nuclei. It must be noted, some heavy nuclei (eg. 240Pu or 252Cf) exhibit fission even in the ground state (without externally added excitation energy). This phenomena is known as the spontaneous fission. This process occur without the addition of the critical energy by the quantum-mechanical process of quantum tunneling through the Coulomb barrier (similarly like alpha particles in the alpha decay). The spontaneous fission contributes to ensure sufficient neutron flux on source range detectors when reactor is subcritical in long term shutdown.
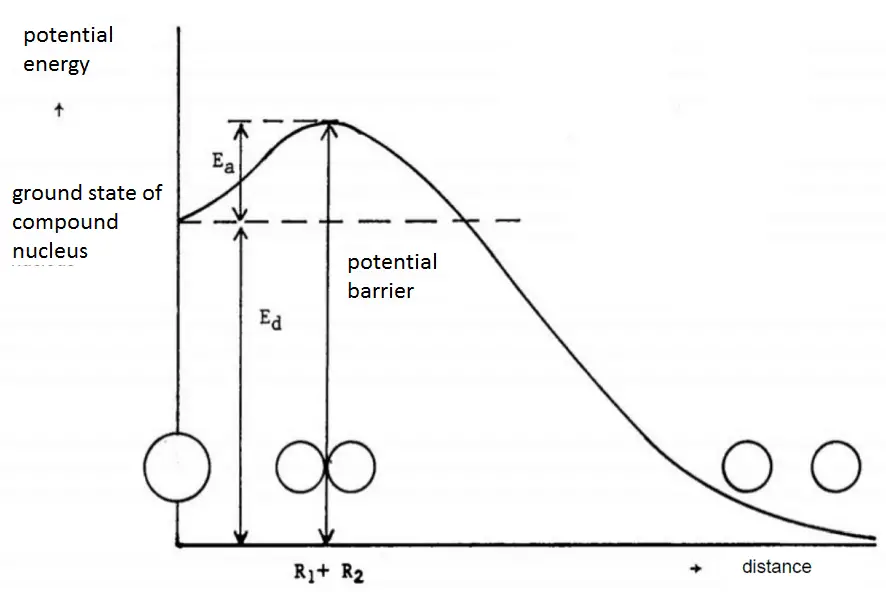
The amount of excitation energy required for fission to occur can be estimated from the magnitude of the electrostatic potential barrier and the dissociation energy of the fission.
In the figure, it is considered the potential energy of the fissioning nucleus as a function of the distance r between the two separating lobes. In order to deform the nucleus into a dumbbell shape, the sufficient kinetic or binding energy must be added to the system. This is due to the fact, nucleons attract one another and energy is required to increase the average distance between them. After the energy is added, the resulting nucleus is in an intermediate state with a larger potential energy than was the potential energy of original nucleus. The height of the potential barrier may be approximated given by:
Ec = Z1.Z2.e2 / (4.π.ε0.(R1+R2))
where R1 and R2 are the respective nuclear radii and ε0 is the permittivity of the vacuum. If this point is reached, the two lobes of the dumbbell begin to separate.
The dissociation energy Ed is equal to the difference between the binding energy of the compound nucleus to be fissioned and the sum of the binding energies of the fission fragments. The minimum activation energy Ea that has to be added to a nucleus to cause fission reaction is thus Ec – Ed.
 The minimum excitation energy required for fission to occur is known as the critical energy (Ec) or threshold energy.The activation energy Ea of nuclides with mass numbers below about 230 is very large, spontaneous fission of these nuclides does not occur. On the other hand nuclei with atomic numbers A > 260 have negative activation energies, so that these nuclei must undergo a decay or they can undergo spontaneous fission. The following table shows critical energies compared to binding energies of the last neutron of a number of nuclei.
The minimum excitation energy required for fission to occur is known as the critical energy (Ec) or threshold energy.The activation energy Ea of nuclides with mass numbers below about 230 is very large, spontaneous fission of these nuclides does not occur. On the other hand nuclei with atomic numbers A > 260 have negative activation energies, so that these nuclei must undergo a decay or they can undergo spontaneous fission. The following table shows critical energies compared to binding energies of the last neutron of a number of nuclei.
All fissioning nuclei do not split in the same way. Although the mass of the initial nucleus is well defined in a reaction, the masses of resulting fission fragments are not. This is the reason there is no single Q-value, but what is usually referred to as the fission Q-value is actually an average of Q-values over all ways of fission.
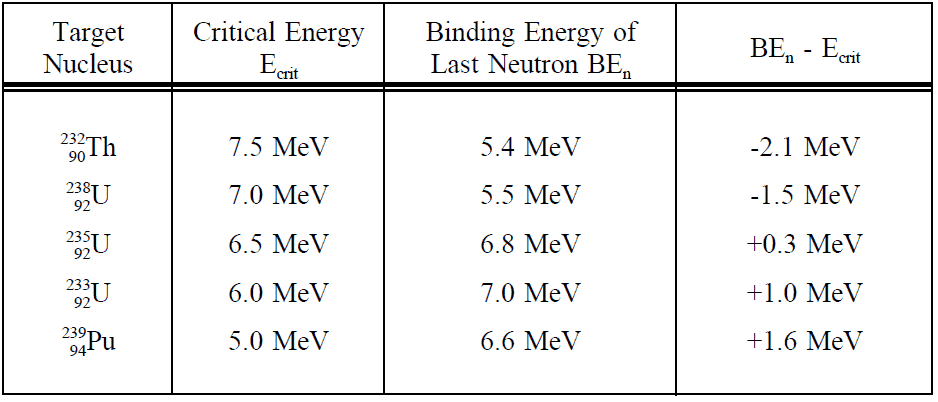 This table shows critical energies compared to binding energies of the last neutron of a number of nuclei.For nuclei lighter than uranium the critical energies are considerably higher (e.g. Ec ~ 20MeV for 208Pb). This is the reason only the heaviest nuclei are of importance in nuclear engineering.
This table shows critical energies compared to binding energies of the last neutron of a number of nuclei.For nuclei lighter than uranium the critical energies are considerably higher (e.g. Ec ~ 20MeV for 208Pb). This is the reason only the heaviest nuclei are of importance in nuclear engineering.
The excitation energy can be added to a nucleus by a neutron, but it is not the only way, how to induce fission. The excitation energy can be added also by bombardment with photons (photofission) or charged particles. In reactor engineering, the most attractive method of causing fission, however, is by forming a compound nucleus with the aid of a neutron.
In the case of neutron-induced fission reactions, an incident neutron provides additional energy to a target nucleus in the form of the kinetic energy and the nuclear binding energy. Neutrons have the principal advantage, they do not need to overcome the coulomb forces as in the case of charged particles.
It can be seen from the table that for fission of 238U or 232Th the neutron must have some additional kinetic energy (negative BEn – Ecrit value), while absorption of a neutron without kinetic energy can already cause fission of 235U (or 233U, 239Pu). For example, according to the table , the binding energy of the last neutron in 236U is 6.8 MeV (target nucleus is 235U), while the critical energy is only 6.5 MeV. Thus, when a thermal neutron is absorbed by 235U, the compound nucleus 236U is produced at about 0.3 MeV above the critical energy and the nucleus splits immediately. Nuclei such as 235U that lead to fission following the absorption of thermal neutron are called fissile nuclei.
For heavy nuclides with atomic number of higher than 90, most of fissile isotopes meet the fissile rule:
Fissile isotopes have 2 x Z – N = 43 ± 2 (example for 235U: 2 x 92 – 143 = 41)
where Z is number of protons and N is number of neutrons.
In general, the heavy nuclei with an odd number of neutrons (235U, 233U, 239Pu and 241Pu) can easily be split, because the neutron that is absorbed to form a compound nucleus with these nuclei is an ‘even’ neutron, so that the binding energy due to the pairing effect is large.
On the other hand, the heavy nuclei with an even number of neutrons (232Th, 238U, 240Pu and 242Pu) have a threshold energy (the kinetic energy) for fission by neutrons, because the absorbed neutron is an ‘odd’ neutron and this neutron makes relatively little binding energy available. For these nuclides fission is thus a threshold reaction.
For example, according to the table, the binding energy of the last neutron in 239U is only 5.5 MeV (target nucleus is 238U), while the critical energy is 7.0 MeV. Thus, when a thermal neutron is absorbed by 238U, fission cannot occur. In order to cause fission of 238U, the incident neutron must have additional kinetic energy. Nuclei such as 238U that lead to fission following the absorption of fast neutron are called fissionable nuclei. It must be noted, also a 208Pb nucleus may be fissioned when struck by a high energy neutron of about 20MeV, but this nucleus is not ordinarily said to be fissionable.
When a nucleus is excited above the potential barrier, it is not sure that fission will occur. Most of absorption reactions result in fission reaction (σf = 585 barns), but a minority results in radiative capture forming 236U. The radiative capture is a reaction, in which the compound nucleus decays to its ground state by gamma emission.The cross-section for radiative capture for thermal neutrons is about 99 barns (for 0.0253 eV neutron). Therefore about 15% of all absorption reactions result in radiative capture of neutron. About 85% of all absorption reactions result in fission.
See also: Uranium 235 Fission
See also: Plutonium 239 Fission
We hope, this article, Critical Energy – Threshold Energy for Fission, helps you. If so, give us a like in the sidebar. Main purpose of this website is to help the public to learn some interesting and important information about radiation and dosimeters.
